The Littlest Big Bang

Scientists are building ultra-cold systems that mimic the most extreme edges of the universe. Can these analogues help solve the big bang’s mysteries?

By James Owen Weatherall Posted 05.07.2008 at 3:30 pm 9 Comments

Mini Fridge The liquid helium in this tube, cooled to just 0.0003°F above absolute zero, shares surprising similarities with the early universe. Jonathan Worth

View Photo Gallery

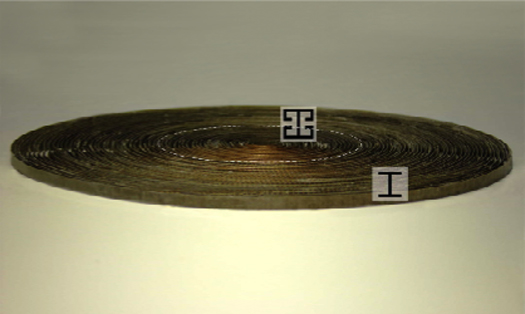
The device is a cylinder a bit smaller than a pinky finger, filled with helium and cooled to just above absolute zero. Inside, a young universe—or something very much like one—evolves. As the helium sloshes about, it mimics a process that may have powered our own universe a few moments after the big bang. And once the fluid settles down, the little whirlpools that remain may be akin to the defects in early spacetime that ultimately gave rise to galaxies, stars and planets.

This universe in a teacup was built by a team at Lancaster University in England. Their study, reported in the January issue of the journal Nature Physics, probed the theory of cosmological inflation. This theory posits that just after the big bang, the universe suddenly grew very rapidly for a split second and then, just as suddenly, slowed down. This faster-than-light expansion is supposed to explain all sorts of things about the universe today, such as why it seems to be more or less the same in every direction, and how large objects, such as clusters of galaxies, coalesced out of the cosmos.

Most physicists believe that inflation occurred. The trouble is in the details—no one knows why it happened, nor quite how. It takes an awful lot of energy to make a universe accelerate, and pretty powerful brakes to get it to slow down again. Plenty of physicists have hazarded guesses at where all that energy came from, but with only one universe around to look at, and with the period of inflation 13 billion years or so in the past, it’s tough to say which of the proposals are right.

Previous Article: The Physics of Beer Pong

Next Article: Broadband over Power Lines

Want to learn more about breakthroughs in electronics, medicine, nanotech, and more?
Subscribe to Popular Science and enter to win $5,000!

9 Comments

link to this comment

fromagesfondus

05/08/08 at 10:10 am

This quandtum stuff is very interesting but remains very confusing to me. Good article though,

link to this comment

jmnowell

05/09/08 at 1:02 am

I think you mean 0.0003 K above absolute zero. At about 4 K, liquid helium has a boiling point well below 0.0003 F, which is around 255 K, where it would only exist in its gaseous state.

link to this comment

Toomanytoys

05/09/08 at 12:15 pm

In the article, the author writes: "The trouble is in the details—no one knows why it happened, nor quite how. It takes an awful lot of energy to make a universe accelerate, and pretty powerful brakes to get it to slow down again."

The answer is simple. God did it. He knows why and how it happened.

Just kidding! I would be a fool to try to explain science with religion or try to support religion with science.

Religion is "Faith".

link to this comment

pernicious

05/09/08 at 1:39 pm

could someone please explain to me how it is they "watched" this process as stated in the article? also, as i understand it, wouldn't their observation of the experiment actually change the results and also render it different from the true Big Bang which one can only assume was absent of the "observer"?

link to this comment

paulkruger

05/09/08 at 2:15 pm

Imagine the original big bang as a tiny singularity and look at it today. Then imagine an experiment like this gone wrong.

A new "little bang" as a singularity. Of course that would fit in the space of this little gadget. But how big and how fast would it grow if the results were similar to the real thing?

Obvious lack of mass aside...this could make for a chilling SciFi story, right?

link to this comment

philbert

05/09/08 at 4:54 pm

jmnowell said:
> I think you mean 0.0003 K above absolute zero. At about 4 K, liquid helium has a boiling point well below 0.0003 F, which is around 255 K, where it would only exist in its gaseous state.

0 °F is about 255 K, as you say. Absolute zero is 0 K, or about -460 °F. So 0.0003 °F above absolute zero is still very nearly -460 °F.

link to this comment

librtyship

05/09/08 at 6:43 pm

Yes things do get more and more complex the deeper we go. For instance Darwin theory seemed pretty reasonable as individual live cells in the body were assumed to be litle gelatinous globs. Now it has been discovered that they contain millions of intricate tiny components which means that each one is a complete tiny factory, then all these cells make up a human body which is an organizm of mind boggling complexity which renders the theory of evolution totally invalid and has to lead to the conclusion that life is created by an extremely high intelligence that we really can't grasp with our limited mental capabilities, then we also have all the other wonders of the universe to ponder, yes Toomanytoys had the right answer, all of these marvels were indeed the handiwork of God and he understands us and understands all else in the universe as he is the creator.

link to this comment

pastkev

05/09/08 at 10:17 pm

It would be really nice to hear about evidence without the spin of religious atheists. Atheism is just as much a religion as anyone who believes in God. Atheism is based on faith and cannot be proved.

It is fine to have a worldview, but please keep your worldview out of the science, it has no place in real science and is offensive to those interested in the facts to have to hear your worldview about them.

Evidence is evidence, let it speak for itself and leave your religious worldview to discuss with your friends who will probably think you are smart; the rest of us do not care.

As he said in Dragnet, just the facts please.

link to this comment

Alex R.

05/16/08 at 6:27 pm

"Evidence" is a very twistable term to use, as it can go more than one way.

Atheism is like a religion! It implies faith in supposed "evidence", does'nt it?